

When you hear a pyramid, it's usually assumed to be a regular square pyramid. A = π × r × √(r² + h²) + π × r² given r and h.Ī pyramid is a 3D solid with a polygonal base and triangular lateral faces.A = A(lateral) + A(base) = π × r × s + π × r² given r and s or.Finally, add the areas of the base and the lateral part to find the final formula for the surface area of a cone:.Thus, the lateral surface area formula looks as follows: R² + h²= s² so taking the square root we got s = √(r² + h²) But that's not a problem at all! We can easily transform the formula using Pythagorean theorem: Usually, we don't have the s value given but h, which is the cone's height.(sector area) = (π × s²) × (2 × π × r) / (2 × π × s)įor finding the missing term of this ratio, you can try out our ratio calculator, too!

(sector area) / (large circle area) = (arc length) / (large circle circumference) so: The formula can be obtained from proportions, as the ratio of the areas of the shapes is the same as the ratio of the arc length to the circumference:
The area of a sector - which is our lateral surface of a cone - is given by the formula:Ī(lateral) = (s × (arc length)) / 2 = (s × 2 × π × r) / 2 = π × r × s The arc length of the sector is equal to 2 × π × r. It's a circular sector, which is the part of a circle with radius s ( s is the cone's slant height).įor the circle with radius s, the circumference is equal to 2 × π × s. Let's have a look at this step-by-step derivation: The base is again the area of a circle A(base) = π × r², but the lateral surface area origins maybe not so obvious:
A = A(lateral) + A(base), as we have only one base, in contrast to a cylinder.We may split the surface area of a cone into two parts:
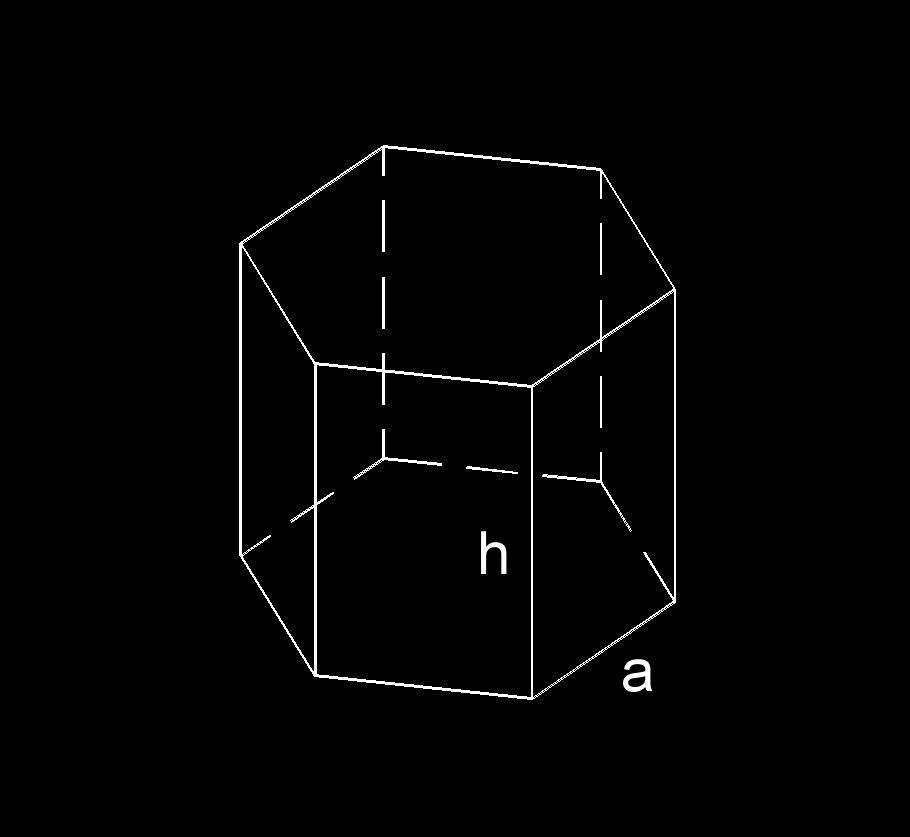
Area of a hexagonal prism how to#
Surface area of a pyramid: A = l × √(l² + 4 × h²) + l², where l is a side length of the square base and h is a height of a pyramid.īut where do those formulas come from? How to find the surface area of the basic 3D shapes? Keep reading, and you'll find out! Surface area of a triangular prism: A = 0.5 × √((a + b + c) × (-a + b + c) × (a - b + c) × (a + b - c)) + h × (a + b + c), where a, b and c are the lengths of three sides of the triangular prism base and h is a height (length) of the prism. Surface area of a rectangular prism (box): A = 2(ab + bc + ac), where a, b and c are the lengths of three sides of the cuboid. Surface area of a cone: A = πr² + πr√(r² + h²), where r is the radius and h is the height of the cone. Surface area of a cylinder: A = 2πr² + 2πrh, where r is the radius and h is the height of the cylinder. Surface area of a cube: A = 6a², where a is the side length. Surface area of a sphere: A = 4πr², where r stands for the radius of the sphere. The formula depends on the type of solid. * n32 symmetry mutation of omnitruncated tilings: 4.6.Our surface area calculator can find the surface area of seven different solids. For p 6, they are tilings of the hyperbolic plane, starting with the truncated triheptagonal tiling. This polyhedron can be considered a member of a sequence of uniform patterns with vertex figure (4.6.2p) and Coxeter-Dynkin diagram. Related polyhedra and tilings Uniform hexagonal dihedral spherical polyhedra It also exists as cells of a number of four-dimensional uniform 4-polytopes, including: Rhombitriangular-hexagonal prismatic honeycomb Snub triangular-hexagonal prismatic honeycomb It exists as cells of four prismatic uniform convex honeycombs in 3 dimensions: The topology of a uniform hexagonal prism can have geometric variations of lower symmetry, including: It can be seen as a truncated hexagonal hosohedron, represented by Schläfli symbol t. If faces are all regular, the hexagonal prism is a semiregular polyhedron, more generally, a uniform polyhedron, and the fourth in an infinite set of prisms formed by square sides and two regular polygon caps. As a semiregular (or uniform) polyhedron Because of the ambiguity of the term octahedron and tilarity of the various eight-sided figures, the term is rarely used without clarification.īefore sharpening, many pencils take the shape of a long hexagonal prism. However, the term octahedron is primarily used to refer to the regular octahedron, which has eight triangular faces. Since it has 8 faces, it is an octahedron. Prisms are polyhedrons this polyhedron has 8 faces, 18 edges, and 12 vertices. In geometry, the hexagonal prism is a prism with hexagonal base. Prism with a 6-sided base Uniform hexagonal prism


 0 kommentar(er)
0 kommentar(er)
